
Mathematik-Online-Aufgabensammlung:

|
[Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen] |
|
Mathematik-Online-Aufgabensammlung: | |
Aufgabe 1178: Orthonormalbasis |
| A B C D E F G H I J K L M N O P Q R S T U V W X Y Z |
Im
![]() ist die Basis
ist die Basis
![]() mit
mit
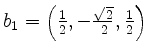 ,
,
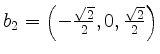 ,
,
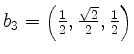
gegeben und ![]() bezeichne die Standardbasis.
bezeichne die Standardbasis.
Die lineare Abbildung ![]() ist durch
ist durch
 ,
,
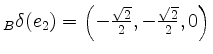 ,
,
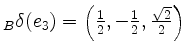
definiert.
Lösung:
| automatisch erstellt am 12. 8. 2008 |