
Mathematik-Online-Kurs: Prüfungsvorbereitung HM 1/2 SS08 - Lösungen zur Probeklausur 4

|
[Home] [Lexikon] [Aufgaben] [Tests] [Kurse] [Begleitmaterial] [Hinweise] [Mitwirkende] [Publikationen] |
|
Mathematik-Online-Kurs: Prüfungsvorbereitung HM 1/2 SS08 - Lösungen zur Probeklausur 4 | |
Aufgabe 1 |
| [vorangehende Seite] [nachfolgende Seite] | [Gesamtverzeichnis][Seitenübersicht] |
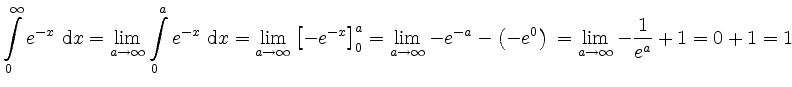
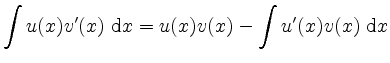
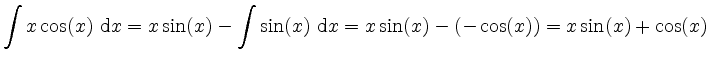
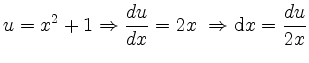
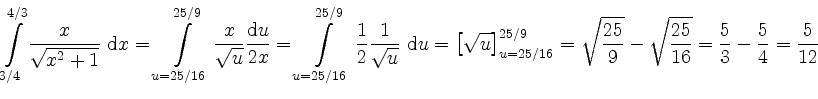
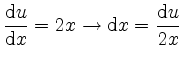


| [vorangehende Seite] [nachfolgende Seite] | [Gesamtverzeichnis][Seitenübersicht] |
| automatisch erstellt am 14.7.2008 |